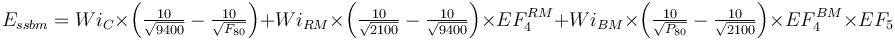Difference between revisions of "Model:Bond-Rowland SSBM"
(→Bond Single Stage Ball Mill Model) |
(→Bond Single Stage Ball Mill Model) |
||
| Line 2: | Line 2: | ||
[[category:Specific Energy Models]] |
[[category:Specific Energy Models]] |
||
[[Category: Bond SSBM Model]] |
[[Category: Bond SSBM Model]] |
||
| − | == Bond Single Stage Ball Mill Model == |
+ | == Bond/Rowland Single Stage Ball Mill Model == |
This is a multi-stage crushing plant feeding a ball mill model that estimates the specific energy consumption (E<sub>BM</sub>) using the Rowland interpretation of the classical Bond work index equation including Rowland efficiency factors. |
This is a multi-stage crushing plant feeding a ball mill model that estimates the specific energy consumption (E<sub>BM</sub>) using the Rowland interpretation of the classical Bond work index equation including Rowland efficiency factors. |
||
| Line 12: | Line 12: | ||
| − | The rod mill oversize feed factor (EF<sub>4</sub><sup>RM</sup>) is calculated using the greater of the sample's rod mill or crushing work index. The optimal feed size and EF<sub>4</sub> are both calculated using whichever is greater. |
+ | The rod mill oversize feed factor (EF<sub>4</sub><sup>RM</sup>) is calculated using the greater of the sample's rod mill or crushing work index (which is usually the Wi<sub>RM</sub>). The optimal feed size and EF<sub>4</sub> are both calculated using whichever is greater. |
| + | |||
| + | <math> |
||
| + | EF_4^{RM} =\left [ 1 + \left( \frac{ (0.907 \times Wi_{RM} - 7) }{ \left (\frac{F_{80} }{ P_{80}} \right )} \right) \left ( {\frac{F_{80} }{ { 16 000 \left(\frac{14.33}{Wi_{RM} } \right )^{0.5}}} }-1 \right ) \right ] |
||
| + | </math> |
||
| + | |||
| + | where: |
||
| + | * F<sub>80</sub> = 9400 µm |
||
| + | * P<sub>80</sub> = 2100 µm |
||
The ball mill oversize feed factor (EF<sub>4</sub><sup>BM</sup>) is always calculated with the ball mill work index. The optimal feed size for the EF<sub>4</sub><sup>BM</sup> is always calculated using the rod mill work index. |
The ball mill oversize feed factor (EF<sub>4</sub><sup>BM</sup>) is always calculated with the ball mill work index. The optimal feed size for the EF<sub>4</sub><sup>BM</sup> is always calculated using the rod mill work index. |
||
| + | |||
| + | <math> |
||
| + | EF_4^{BM} =\left [ 1 + \left( \frac{(0.907 \times Wi_{BM} - 7) }{ \left (\frac{F_{80} }{ P_{80}} \right )} \right) \left ( {\frac{F_{80} }{ { 4000 \left(\frac{14.33}{Wi_{RM} } \right )^{0.5}}} }-1 \right ) \right ] |
||
| + | </math> |
||
| + | |||
| + | where: |
||
| + | * F<sub>80</sub> = 2100 µm |
||
| + | * P<sub>80</sub> = the specified circuit product size, µm |
||
== Default parameter values == |
== Default parameter values == |
||
Revision as of 15:02, 23 April 2014
Bond/Rowland Single Stage Ball Mill Model
This is a multi-stage crushing plant feeding a ball mill model that estimates the specific energy consumption (EBM) using the Rowland interpretation of the classical Bond work index equation including Rowland efficiency factors.
The rod mill oversize feed factor (EF4RM) is calculated using the greater of the sample's rod mill or crushing work index (which is usually the WiRM). The optimal feed size and EF4 are both calculated using whichever is greater.
![EF_4^{RM} =\left [ 1 + \left( \frac{ (0.907 \times Wi_{RM} - 7) }{ \left (\frac{F_{80} }{ P_{80}} \right )} \right) \left ( {\frac{F_{80} }{ { 16 000 \left(\frac{14.33}{Wi_{RM} } \right )^{0.5}}} }-1 \right ) \right ]](/images/math/7/e/9/7e9b49234a38249bd7b36477cdc0e3ef.png)
where:
- F80 = 9400 µm
- P80 = 2100 µm
The ball mill oversize feed factor (EF4BM) is always calculated with the ball mill work index. The optimal feed size for the EF4BM is always calculated using the rod mill work index.
![EF_4^{BM} =\left [ 1 + \left( \frac{(0.907 \times Wi_{BM} - 7) }{ \left (\frac{F_{80} }{ P_{80}} \right )} \right) \left ( {\frac{F_{80} }{ { 4000 \left(\frac{14.33}{Wi_{RM} } \right )^{0.5}}} }-1 \right ) \right ]](/images/math/a/1/a/a1a63ddfd19337a26911b8e6354d94b3.png)
where:
- F80 = 2100 µm
- P80 = the specified circuit product size, µm
Default parameter values
- Assumed crusher product P80 size, µm = 9400
- Hypothetical rod mill product size, µm = 2100 (mid-point of the range between typical rod mill work index test P80 and typical ball mill work index test F80).