Difference between revisions of "Model:Raw Bond/Barratt SAB & SABC Model"
(→Raw Bond Model) |
(→Default parameter values) |
||
| Line 22: | Line 22: | ||
== Default parameter values == |
== Default parameter values == |
||
* Maximum transfer size, µm = 6000 |
* Maximum transfer size, µm = 6000 |
||
| − | * Minimum transfer size, µm = 400 [[Bibliography: Specific energy consumption models|<sup>[Starkey et al.]</sup>]] |
+ | * Minimum transfer size, µm = 400 [[Bibliography: Specific energy consumption models|<sup>[Starkey et al. 2008]</sup>]] |
| + | * Hypothetical tertiary crusher product size, µm = 18850 [[Bibliography: Specific energy consumption models|<sup>[Barratt 1979]</sup>]] |
||
| + | * Hypothetical rod mill product size, µm = 2100 (mid-point of the range between typical rod mill work index test P<sub>80</sub> and typical ball mill work index test F<sub>80</sub>). |
||
Revision as of 21:40, 6 November 2012
Raw Bond Model
This is a SAG or AG mill plus ball mill model that estimates the specific energy consumption (ESAG) using the 1979 Barratt equation and the classical Bond work index equation including Rowland efficiency factors. The model allows a manual calibration factor on both equations (CFSAG and CFball, respectively).
This formula usually gives higher total circuit specific energy consumption estimates than the other Bond/Barratt models. This is largely due to the absence of a phantom cyclone effect in the equations.
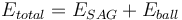
As with most models, the power split between the SAG and ball mills is evaluated and the transfer size (T80) necessary to balance the power draw between the two stages is estimated. If that transfer size is within the specified limits, then the calculation proceeds to calculate the ESAG and Eball using that transfer size. If the transfer size to balance the power is outside the specified limits, then the transfer size size is forced to the limit and the ESAG and Eball values are then calculated. SAG-limited circuits (where the T80 hits the upper limit) will result in the ball mill over-grinding unless (in the case of a variable speed mill) the ball mill speed is reduced to control the grind size. Ball-limited circuits (where the T80 hits the lower limit) will result in the charge level in the SAG mill dropping until its power draw matches the throughput limit dictated by the ball mill. It is important to realize that once the SAG mill charge level drops below
Circuits that operate at or near the transfer size limits should be less efficient than the default formulas will estimate. If you have a design that is consistently operating around the limits, then consider adding a contingency to the power estimates to account for this poor grinding efficiency. Alternatively, if you are designing a new circuit then choose a different combination of SAG and ball mills that operates in a more reasonable transfer size range.
Recommended Usage
This model should be the upper end-member of the range of Bond/Barratt style models. It is useful when interpreting pilot plant results to determine where the pilot plant results fall relative to the spectrum of models. It can also be used as an extra-conservative model for design work.
Because this model does not include an internal phantom cyclone mechanism, it is customary to include an allowance for it in the CFball factor by using a value of 0.95 (some fines in SAG product) to 0.85 (many fines in SAG product). If a SAG product does not include extra fines versus a standard size distribution, then use a value of 1.00.
Default parameter values
- Maximum transfer size, µm = 6000
- Minimum transfer size, µm = 400 [Starkey et al. 2008]
- Hypothetical tertiary crusher product size, µm = 18850 [Barratt 1979]
- Hypothetical rod mill product size, µm = 2100 (mid-point of the range between typical rod mill work index test P80 and typical ball mill work index test F80).

