Difference between revisions of "Benchmarking: Amelunxen SGI - Agnico Eagle"
(→Modelling) |
(→Results) |
||
| (13 intermediate revisions by one other user not shown) | |||
| Line 6: | Line 6: | ||
Survey conducted shortly after start-up |
Survey conducted shortly after start-up |
||
| + | |||
| + | Actual operating data, table 7 from the reference |
||
| + | * Throughput = 210 dry tonnes per hour |
||
| + | * SAG circuit F<sub>80</sub> = 100 mm |
||
| + | * transfer T<sub>80</sub> = 244 µm |
||
| + | * circuit P<sub>80</sub> = 74 µm |
||
| + | * SAG power draw (at motor input) = 1350 kW (at mill shell = 1283 kW) |
||
| + | * SAG mill ball charge = 10.6 % vol |
||
| + | * SAG mill discharge % solids = 84% weight |
||
| + | * SAG mill speed 8.59 RPM (53.4% of critical) |
||
| + | * E<sub>SAG</sub> at mill shell = 1283/210 = 6.11 kWh/t |
||
| + | (to achieve DCS power of 1350 kW requires 14% vol mill load) |
||
| + | * ball mill power draw (sum of 2 mills, at motor input) = 1422 kW (at mill shell = 2 × 674 kW = 1348 kW) |
||
| + | * ball mill % solids = 83% weight |
||
| + | (to achieve 1424 kW, assume 30% vol mill charge, 17 RPM) |
||
| + | * E<sub>bm</sub> at mill shell = 1348/210 = 6.41 kWh/t |
||
| + | * ball mill operating Wi<sub>O</sub> relative to motor input = 13.0 kWh/t (= 13.7 kWh/t relative to mill shell) |
||
==Design Criteria== |
==Design Criteria== |
||
| + | * SGI (actually SPI™) of ore (Oct 1, 2000 to - April 30,2001); assumed to be the 25<sup>th</sup> percentile = 24 minutes. |
||
| − | Actual operating data from Esperanza |
||
| + | * Wi<sub>BM</sub> assumed to be 10 kWh/tonne. |
||
| − | * A×b of Esperanza ore (Aug-Dec 2012); avg= 39; range is 30.7 to 45.5. |
||
| + | * Plant availability = 94.4% |
||
| − | * Wi<sub>BM</sub> average is 16.0 kWh/st, = 17.6 kWh/tonne. |
||
| − | * E<sub>SAG</sub> for "normal" blasting, average = 5.5 kWh/tonne; range is 3.9 to 7.2 kWh/tonne. |
||
| − | * E<sub>SAG</sub> for "high intensity" blasting typically 10% lower E<sub>SAG</sub>. |
||
| − | * SAG feed size range 33.6% to 49.0 percent passing 1¼ inch (32 mm). |
||
| − | |||
| − | Design criteria for expansion cases (which may reflect Esperanza operating conditions) |
||
| − | * SAG circuit F<sub>80</sub> = 132 mm |
||
| − | * transfer T<sub>80</sub> = 3500 µm (text erroneously says "3500 mm") |
||
| − | * BM circuit P<sub>80</sub> = 180 µm |
||
| − | * SAG mill power utilization = 92.3% |
||
| − | * Ball mill power utilization = 95% |
||
| − | * Plant availability = 93% |
||
Mill criteria |
Mill criteria |
||
| − | * SAG mill: |
+ | * SAG mill: 24 foot nom diam by 12 foot effective grinding length (EGL) |
| − | * ball mill: |
+ | * ball mill: two 11 foot nom diam by 17 foot (assumed to be EGL) |
| − | * SAG mill |
+ | * SAG mill single variable speed motor 4500 HP |
| − | * Ball mill |
+ | * Ball mill single fixed speed motors 1000 HP (each mill) |
| + | * assume rubber liners in SAG, nominal 8½ inch liner thickness |
||
| − | (some references give 30,000 HP for SAG motor; this is thought to be the value excluding a 5% design margin) |
||
| + | * assume steel liners in ball mills, nominal 3¼ inch liner thickness |
||
==Modelling== |
==Modelling== |
||
| + | The Amelunxen SGI model for SAB circuit is used. |
||
| − | The Bond/Barratt SABC-B model is used with the default 10% E<sub>ssbm</sub> calibration factor. |
||
| − | * circuit F<sub>80</sub> = |
+ | * circuit F<sub>80</sub> = 100 mm |
| − | * circuit P<sub>80</sub> = |
+ | * circuit P<sub>80</sub> = 74 µm |
| − | * The average ball mill work index given as 16 kWh/short ton = 17.6 kWh/tonne |
||
| − | * The relationship between A×b and Wi<sub>RM</sub> for Andean copper porphyries can be used to determine the following rod mill work index values. |
||
| − | ** A×b of Esperanza ore; avg= 39; becomes Wi<sub>RM</sub>=14.5 kWh/tonne |
||
| − | * No crushing work index is given. Assume a value of 12 kWh/tonne (slightly higher than typical porphyry) |
||
| − | * No ore density is given. Assume similar to Los Bronces, density=2.64 t/m³ |
||
SAG mill is modelled using following: |
SAG mill is modelled using following: |
||
| − | * |
+ | * full Morrell SAG grate C-model |
| − | * assume ore density 2. |
+ | * assume ore density 2.7 t/m³ |
| + | * effective dimensions 6882 mm diam × 3657 mm EGL |
||
| − | * nominal diameter 40 foot, effective grinding length 26 foot (deduct 2 ft from cylinder length) |
||
| − | * assume 6 inch liner effective thickness, mill speed 75% of critical |
||
| − | * ball charge 16% v/v; total filling 26% v/v gives the desired 92.4% utilization of motor power |
||
Ball mill is modelled using following: |
Ball mill is modelled using following: |
||
| − | * |
+ | * full Morrell ball mill wet overflow C-model |
| − | * assume ore density 2. |
+ | * assume ore density 2.7 t/m³ |
| + | * effective dimensions 3186 mm diam × 5181 mm EGL |
||
| − | * nominal diameter 27 foot, effective grinding length 44.5 foot (deduct 6 inches from cylinder length) |
||
| + | |||
| − | * assume 6 inch liner effective thickness, mill speed 75% of critical |
||
| + | The model defaults are used for: |
||
| − | * total filling 30% v/v gives the desired 95% utilization of motor power |
||
| + | * CF<sub>SAG</sub> = 1.0 (normal feed size, no pebble crushing) |
||
| + | * CF<sub>ball</sub> = 1.10 (relationship for Los Bronces in Amelunxen et al, 2013) |
||
== Results == |
== Results == |
||
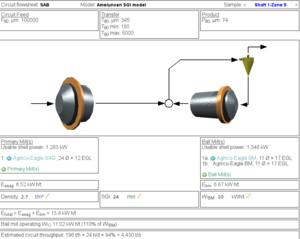
| + | [[file:Benchmarking-AgnicoEagle.png|thumb|right| Screenshot of Agnico Eagle circuit]] |
||
| + | Result for default Amelunxen SGI SAB model conditions: |
||
| + | {| class="wikitable" border="1" |
||
| + | |- |
||
| + | ! |
||
| + | !Esag |
||
| + | !Epeb |
||
| + | !Ebm |
||
| + | !Etotal |
||
| + | !t/h |
||
| + | |- |
||
| + | | Predicted |
||
| + | | 6.5 |
||
| + | | - |
||
| + | | 6.9 |
||
| + | | 13.4 |
||
| + | | 196 |
||
| + | |- |
||
| + | | Survey |
||
| + | | 6.1 |
||
| + | | - |
||
| + | | 6.4 |
||
| + | | 12.5 |
||
| + | | 210 |
||
| + | |- |
||
| + | | Difference |
||
| + | | 0.4 |
||
| + | | - |
||
| + | | 0.5 |
||
| + | | 0.9 |
||
| + | | 14 |
||
| + | |- |
||
| + | | Difference |
||
| + | | model 7% high |
||
| + | | - |
||
| + | | model 8% high |
||
| + | | model 7% high |
||
| + | | model 7% low |
||
| + | |} |
||
| + | |||
| + | ==Discussion== |
||
| + | The calibration factor for SAG milling, CF<sub>SAG</sub>, is given in Amelunxen et al (2013) as: |
||
| + | * 1.00 for "normal feed size with no pebble crushing", |
||
| + | * 0.90 for "fine SAG feed" |
||
| + | * 0.85 for "pebble crushing" |
||
| + | * 0.77 for both "fine SAG feed" and "pebble crushing" |
||
| + | |||
| + | Back-calculating the CF<sub>SAG</sub> for Laronde ore gives 0.94, mid-way between "normal" and "fine" in the list above. Amelunxen et al (2013) do not actually reveal what the basis is for determining if an ore is "fine", but 100 mm could be on the cusp of being fine or coarse. In reality, the CF<sub>SAG</sub> factor should be a continuous and smooth function of feed size and not a step-change. |
||
| + | |||
| + | The Starkey et al (2001) reference does not give a suitable range of ball mill work index values suitable for determining a 25<sup>th</sup> percentile. The operating work index is given as 12.3 kWh/t (13.0 kWh/t motor input basis). Back-calculating a CF<sub>ball</sub> value is not really possible with the data provided. |
||
| + | |||
| + | |||
| + | If the model is run with a manual CF<sub>SAG</sub> = 0.95, the following results are returned: |
||
| + | Result for Amelunxen SGI SAB model with CF<sub>SAG</sub> = 0.95 |
||
| − | Only one ball mill work index is provided, claimed to be the 'average'. The predictions of the min/max range are dubious because the ball mill work index should change between hard and soft ore. |
||
| + | {| class="wikitable" border="1" |
||
| + | |- |
||
| + | ! |
||
| + | !Esag |
||
| + | !Epeb |
||
| + | !Ebm |
||
| + | !Etotal |
||
| + | !t/h |
||
| + | |- |
||
| + | | Predicted |
||
| + | | 6.4 |
||
| + | | - |
||
| + | | 6.8 |
||
| + | | 13.2 |
||
| + | | 199 |
||
| + | |- |
||
| + | | Survey |
||
| + | | 6.1 |
||
| + | | - |
||
| + | | 6.4 |
||
| + | | 12.5 |
||
| + | | 210 |
||
| + | |- |
||
| + | | Difference |
||
| + | | 0.3 |
||
| + | | - |
||
| + | | 0.4 |
||
| + | | 0.7 |
||
| + | | 11 |
||
| + | |- |
||
| + | | Difference |
||
| + | | model 4.9% high |
||
| + | | - |
||
| + | | model 6.3% high |
||
| + | | model 5.6% high |
||
| + | | model 5.5% low |
||
| + | |} |
||
Latest revision as of 20:04, 22 May 2020
Contents
Benchmarking: Amelunxen SGI Specific Energy Consumption - Agnico Eagle Laronde
Starkey, J., Robitaille, J., Cousin, P., Jordan, J. and Kosick, G., Design of the Agnico-Eagle Laronde Division SAG mill. Proceedings of SAG 2001, pages III-165 to III-178.
Survey conducted shortly after start-up
Actual operating data, table 7 from the reference
- Throughput = 210 dry tonnes per hour
- SAG circuit F80 = 100 mm
- transfer T80 = 244 µm
- circuit P80 = 74 µm
- SAG power draw (at motor input) = 1350 kW (at mill shell = 1283 kW)
- SAG mill ball charge = 10.6 % vol
- SAG mill discharge % solids = 84% weight
- SAG mill speed 8.59 RPM (53.4% of critical)
- ESAG at mill shell = 1283/210 = 6.11 kWh/t
(to achieve DCS power of 1350 kW requires 14% vol mill load)
- ball mill power draw (sum of 2 mills, at motor input) = 1422 kW (at mill shell = 2 × 674 kW = 1348 kW)
- ball mill % solids = 83% weight
(to achieve 1424 kW, assume 30% vol mill charge, 17 RPM)
- Ebm at mill shell = 1348/210 = 6.41 kWh/t
- ball mill operating WiO relative to motor input = 13.0 kWh/t (= 13.7 kWh/t relative to mill shell)
Design Criteria
- SGI (actually SPI™) of ore (Oct 1, 2000 to - April 30,2001); assumed to be the 25th percentile = 24 minutes.
- WiBM assumed to be 10 kWh/tonne.
- Plant availability = 94.4%
Mill criteria
- SAG mill: 24 foot nom diam by 12 foot effective grinding length (EGL)
- ball mill: two 11 foot nom diam by 17 foot (assumed to be EGL)
- SAG mill single variable speed motor 4500 HP
- Ball mill single fixed speed motors 1000 HP (each mill)
- assume rubber liners in SAG, nominal 8½ inch liner thickness
- assume steel liners in ball mills, nominal 3¼ inch liner thickness
Modelling
The Amelunxen SGI model for SAB circuit is used.
- circuit F80 = 100 mm
- circuit P80 = 74 µm
SAG mill is modelled using following:
- full Morrell SAG grate C-model
- assume ore density 2.7 t/m³
- effective dimensions 6882 mm diam × 3657 mm EGL
Ball mill is modelled using following:
- full Morrell ball mill wet overflow C-model
- assume ore density 2.7 t/m³
- effective dimensions 3186 mm diam × 5181 mm EGL
The model defaults are used for:
- CFSAG = 1.0 (normal feed size, no pebble crushing)
- CFball = 1.10 (relationship for Los Bronces in Amelunxen et al, 2013)
Results
Result for default Amelunxen SGI SAB model conditions:
| Esag | Epeb | Ebm | Etotal | t/h | |
|---|---|---|---|---|---|
| Predicted | 6.5 | - | 6.9 | 13.4 | 196 |
| Survey | 6.1 | - | 6.4 | 12.5 | 210 |
| Difference | 0.4 | - | 0.5 | 0.9 | 14 |
| Difference | model 7% high | - | model 8% high | model 7% high | model 7% low |
Discussion
The calibration factor for SAG milling, CFSAG, is given in Amelunxen et al (2013) as:
- 1.00 for "normal feed size with no pebble crushing",
- 0.90 for "fine SAG feed"
- 0.85 for "pebble crushing"
- 0.77 for both "fine SAG feed" and "pebble crushing"
Back-calculating the CFSAG for Laronde ore gives 0.94, mid-way between "normal" and "fine" in the list above. Amelunxen et al (2013) do not actually reveal what the basis is for determining if an ore is "fine", but 100 mm could be on the cusp of being fine or coarse. In reality, the CFSAG factor should be a continuous and smooth function of feed size and not a step-change.
The Starkey et al (2001) reference does not give a suitable range of ball mill work index values suitable for determining a 25th percentile. The operating work index is given as 12.3 kWh/t (13.0 kWh/t motor input basis). Back-calculating a CFball value is not really possible with the data provided.
If the model is run with a manual CFSAG = 0.95, the following results are returned:
Result for Amelunxen SGI SAB model with CFSAG = 0.95
| Esag | Epeb | Ebm | Etotal | t/h | |
|---|---|---|---|---|---|
| Predicted | 6.4 | - | 6.8 | 13.2 | 199 |
| Survey | 6.1 | - | 6.4 | 12.5 | 210 |
| Difference | 0.3 | - | 0.4 | 0.7 | 11 |
| Difference | model 4.9% high | - | model 6.3% high | model 5.6% high | model 5.5% low |